

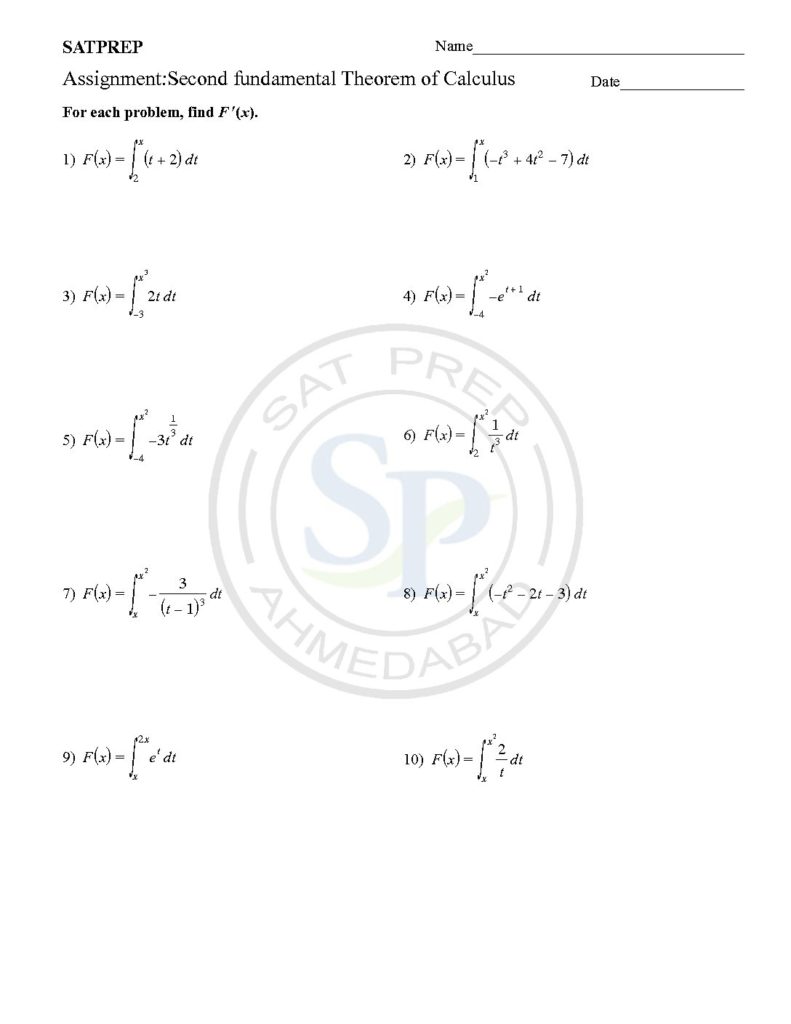
If $F(x)$ is any antiderivative of $f(x)$, then it follows that $F(x) = g(x) + C$ where $C$ is a constant. By the The Fundamental Theorem of Calculus Part 1, we know that $g(x)$ must be an antiderivative of $f(x)$, that is $g'(x) = f(x)$.
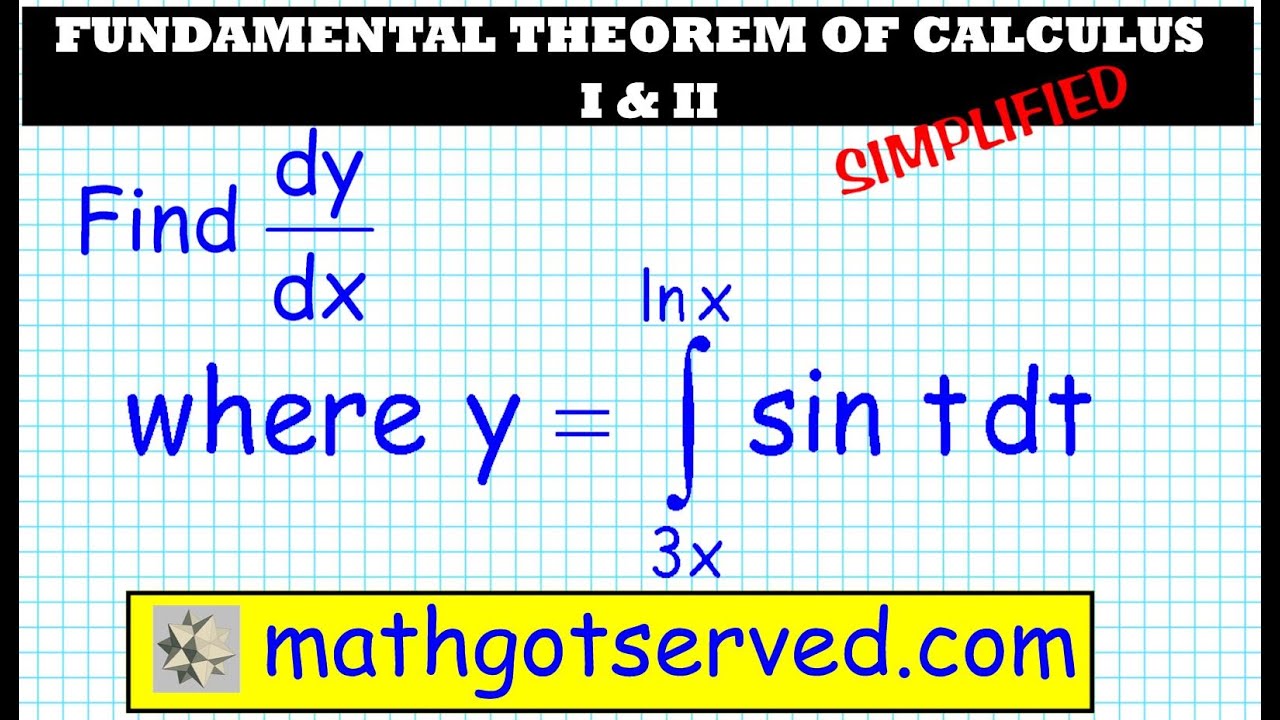

Theorem 1 (The Fundamental Theorem of Calculus Part 2): If a function $f$ is continuous on an interval $$, then it follows that $\int_a^b f(x) \: dx = F(b) - F(a)$, where $F$ is a function such that $F'(x) = f(x)$ ( $F$ is any antiderivative of $f$). We will now look at the second part to the Fundamental Theorem of Calculus which gives us a method for evaluating definite integrals without going through the tedium of evaluating limits. Recall that the The Fundamental Theorem of Calculus Part 1 essentially tells us that integration and differentiation are "inverse" operations. This tells us this: when we evaluate f at n (somewhat) equally spaced points in, the average value of these samples is f ( c ) as n → ∞.The Fundamental Theorem of Calculus Part 2 Lim n → ∞ 1 b - a ∑ i = 1 n f ( c i ) Δ x = 1 b - a ∫ a b f ( x ) d x = f ( c ). = 1 b - a ∑ i = 1 n f ( c i ) Δ x (where Δ x = ( b - a ) / n ) = 1 b - a ∑ i = 1 n f ( c i ) b - a n = ∑ i = 1 n f ( c i ) 1 n ( b - a ) ( b - a ) Multiply this last expression by 1 in the form of ( b - a ) ( b - a ): Consider the function f (x)ex and the points a 0, bln2, cln6. Verify the result by substitution into the equation. The average of the numbers f ( c 1 ), f ( c 2 ), …, f ( c n ) is:ġ n ( f ( c 1 ) + f ( c 2 ) + ⋯ + f ( c n ) ) = 1 n ∑ i = 1 n f ( c i ). A).Use the Fundamental Theorem of Calculus, Part 1, to find the function f that satisfies the equation 0 to x f (t)dt 2cosx+3x2. Next, partition the interval into n equally spaced subintervals, a = x 1 < x 2 < ⋯ < x n + 1 = b and choose any c i in. Viewed 54 times -3 begingroup I have a difficulty in understanding the proof of fundamental theorem of calculus (part 2): intabf(x)dxF(b)-F(a). First, recognize that the Mean Value Theorem can be rewritten asį ( c ) = 1 b - a ∫ a b f ( x ) d x ,įor some value of c in. Question on the Proof about Fundamental Theorem of Calculus (part 2) Ask Question Asked 4 months ago. The value f ( c ) is the average value in another sense. This proves the second part of the Fundamental Theorem of Calculus. The first part of the theorem states that every continuous function has an antiderivative, and every anti-derivative has a derivative. Consequently, it does not matter what value of C we use, and we might as well let C = 0. The Fundamental Theorem of Calculus Part 2 Example 1: Find » ¼ º « ¬ ª³ x t dt dx d 1 3 Example 2: Find » ¼ º « ¬ ª ³ x t dt dx d 3 (2 2) Practice Problem 1: Find » ¼ º « ¬ ª ³ x t dt dx d 2 (3 1) Example 3: Find » ¼. The first part of the theorem says that if we first integrate and then differentiate the result. The Fundamental Theorem of Calculus, Part 2 Warm-up Find the area under the curve f(x) 2x 1 over -1, 1. If f happens to be a positive function, then g (x) can be interpreted as the area under the graph of f from a to x. This means that G ( b ) - G ( a ) = ( F ( b ) + C ) - ( F ( a ) + C ) = F ( b ) - F ( a ), and the formula we’ve just found holds for any antiderivative. Part 1 (FTC1) If f is a continuous function on a, b, then the function g defined by. Furthermore, Theorem 5.1.1 told us that any other antiderivative G differs from F by a constant: G ( x ) = F ( x ) + C. We now see how indefinite integrals and definite integrals are related: we can evaluate a definite integral using antiderivatives. = - ∫ c a f ( t ) d t + ∫ c b f ( t ) d t = ∫ a c f ( t ) d t + ∫ c b f ( t ) d t Using the properties of the definite integral found in Theorem 5.2.1, we know First, let F ( x ) = ∫ c x f ( t ) d t. Suppose we want to compute ∫ a b f ( t ) d t. Consider a function f defined on an open interval containing a, b and c. We have done more than found a complicated way of computing an antiderivative.


 0 kommentar(er)
0 kommentar(er)
